Help with a math question?
2021-02-19 3:00 pm
Hi, I've been struggling with this for a while can someone explain it to me please?

回答 (3)
2021-02-19 5:06 pm
✔ 最佳答案
The answer is as follows: 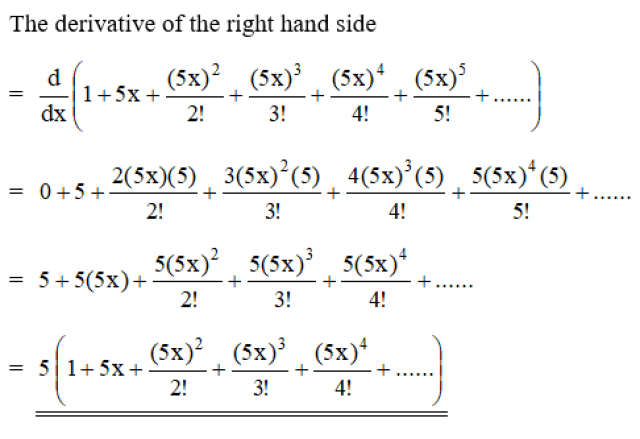
2021-02-19 10:30 pm
Another way is to expand the terms first:
e^(5x) = 1 + 5x + (25/2)x^2 + (125/6)x^3 + (625/24)x^4 + (3125/120)x^5 + (15625/720)x^6 + ...
so that
d[e^(5x)]/dx = 5 + 25x + (125/2)x^2 + (625/6)x^3 + (3125/24)x^4 + ...
e^(5x) = 1 + 5x + (25/2)x^2 + (125/6)x^3 + (625/24)x^4 + (3125/120)x^5 + (15625/720)x^6 + ...
so that
d[e^(5x)]/dx = 5 + 25x + (125/2)x^2 + (625/6)x^3 + (3125/24)x^4 + ...
2021-02-19 3:31 pm
Remember the chain rule? Let u = 5x and:
d/dx u^n = (d/du u^n) * (du/dx)
= n u^(n-1) * du/dx
Plug u=5x back in and note that du/dx = 5
d/dx (5x)^n = 5n * (5x)^(n-1)
The constant term in that sum on the right vanishes and the derivatives of the next 5 terms follow the above pattern. When simplifying, you'll find that n/n! will reduce to 1/(n-1)! in each term, so that you end up 5 times the original series.
...which is just what you should expect from using the chain rule d/dx e^(5x) directly, without expanding the power series.
d/dx u^n = (d/du u^n) * (du/dx)
= n u^(n-1) * du/dx
Plug u=5x back in and note that du/dx = 5
d/dx (5x)^n = 5n * (5x)^(n-1)
The constant term in that sum on the right vanishes and the derivatives of the next 5 terms follow the above pattern. When simplifying, you'll find that n/n! will reduce to 1/(n-1)! in each term, so that you end up 5 times the original series.
...which is just what you should expect from using the chain rule d/dx e^(5x) directly, without expanding the power series.
收錄日期: 2021-04-23 23:08:45
原文連結 [永久失效]:
https://hk.answers.yahoo.com/question/index?qid=20210219070035AAzsIUt


