Geometry(similarity) ?
2020-09-29 11:02 pm
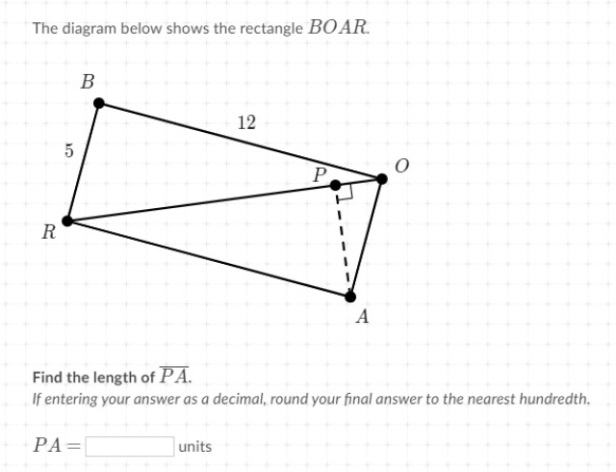
How can I solve for PA on this problem?
回答 (9)
2020-09-30 4:30 am
✔ 最佳答案
OR=sqr(12^2+5^2)=13Tri. AOP ~ Tri. AOR
=>
AP/AR=AO/OR
=>
AP/12=5/13
=>
AP=5(12)/13~4.62.
2020-09-30 12:08 am
For rectangle BOAR:
OA = BR = 5 and BO = RA = 12
In ΔROA:
OR² = OA² + RA² (Pythagorean theorem)
OR = √(5² + 12²)
OR = √13
Area of ΔROA:
(1/2) × PA × OR = (1/2) × OA × RA
(1/2) × PA × 13 = (1/2) × 5 × 12
PA = 5 × 12 / 13
PA = 4.62
OA = BR = 5 and BO = RA = 12
In ΔROA:
OR² = OA² + RA² (Pythagorean theorem)
OR = √(5² + 12²)
OR = √13
Area of ΔROA:
(1/2) × PA × OR = (1/2) × OA × RA
(1/2) × PA × 13 = (1/2) × 5 × 12
PA = 5 × 12 / 13
PA = 4.62
2020-09-30 8:05 pm
By areas. Area of ROA = 30 sq units (5 x 12/2)
base RA = square root 169 = 13,
height is PA, half base = 6.5
30/6.5 = 4.62 units
base RA = square root 169 = 13,
height is PA, half base = 6.5
30/6.5 = 4.62 units
2020-09-30 6:53 pm
PA/OA = 12/OR = 12/(12^2+5^2)^(1/2) = 12/(144+25)^(1/2) = 12/13;
PA =(12/13)OA=(12/13)5=(60/13)=4.615384615=4.62 rounded to nearest hundredth
PA =(12/13)OA=(12/13)5=(60/13)=4.615384615=4.62 rounded to nearest hundredth
2020-09-30 12:25 am
OR=sqrt(12^2+5^2)=13
12/13=.9230
.9230*5= 4.615
12/13=.9230
.9230*5= 4.615
2020-09-29 11:53 pm
RO = 13
The area of ∆ RAO = 30,
RO/2 * PA = 30
PA = 60/13 = 4.62
∆RPA is similar to ∆PAO
OA/RA = PA/RP
5/12 = PA/RP
The area of ∆ RAO = 30,
RO/2 * PA = 30
PA = 60/13 = 4.62
∆RPA is similar to ∆PAO
OA/RA = PA/RP
5/12 = PA/RP
2020-09-29 11:09 pm
Since RO cuts the rectangle in half through the diagonal, ROA and BRO are identical.
So we need the height of the triangle with lengths 5 and 12 when the hypotenuse is the base.
Let's do this. If we put the triangle upright, we have the base of 5 and height of 12. We can find the area:
A = bh/2
A = 5(12)/2
A = 30 unit²
We can use Pythagarean theorem to find the length of the hypotenuse:
a² + b² = c²
5² + 12² = c²
25 + 144 = c²
169 = c²
13 = c
With the same triangle on its hypotenuse for a base, the area is unchanged from when we solved for it above. We can use that information to solve for the unknown height:
A = bh/2
30 = 13h/2
60 = 13h
h = 60/13
h = 4.62 units (rounded to 2DP)
So we need the height of the triangle with lengths 5 and 12 when the hypotenuse is the base.
Let's do this. If we put the triangle upright, we have the base of 5 and height of 12. We can find the area:
A = bh/2
A = 5(12)/2
A = 30 unit²
We can use Pythagarean theorem to find the length of the hypotenuse:
a² + b² = c²
5² + 12² = c²
25 + 144 = c²
169 = c²
13 = c
With the same triangle on its hypotenuse for a base, the area is unchanged from when we solved for it above. We can use that information to solve for the unknown height:
A = bh/2
30 = 13h/2
60 = 13h
h = 60/13
h = 4.62 units (rounded to 2DP)
2020-09-29 11:09 pm
I'm guessing a bit at this one, but OA is 5 (same as BR)
By pythagorean theorem, PO^2 + PA^2 = OA^2,
Thus PO^2 + PA^2 = 25
Ratio of PO to PA is same as the larger triangle (ie same as BR to BO) so 5 to 12
Thus (PO) * 12 = (PA) * 5.
I've gotten you 2 equations, you figure it out from there.
By pythagorean theorem, PO^2 + PA^2 = OA^2,
Thus PO^2 + PA^2 = 25
Ratio of PO to PA is same as the larger triangle (ie same as BR to BO) so 5 to 12
Thus (PO) * 12 = (PA) * 5.
I've gotten you 2 equations, you figure it out from there.
2020-09-30 6:09 pm
Whatever the triangle, the sum of the 3 angles is always 180 °. So in the triangle SQU, you can write:
In the right triangle (ORA):
yellow + red + 90 = 180
yellow = 90 - red
In the right triangle (OPA):
blue + yellow + 90 = 180
blue = 90 - yellow
blue = 90 - (90 - red)
blue = red
AP = AO.cos(blue) → we've just seen that: blue = red
AP = AO.cos(red) → where: cos(red) = AR/OR
AP = AO.(AR/OR)
AP = 5 * (12/13)
AP ≈ 4.615
In the right triangle (ORA):
yellow + red + 90 = 180
yellow = 90 - red
In the right triangle (OPA):
blue + yellow + 90 = 180
blue = 90 - yellow
blue = 90 - (90 - red)
blue = red
AP = AO.cos(blue) → we've just seen that: blue = red
AP = AO.cos(red) → where: cos(red) = AR/OR
AP = AO.(AR/OR)
AP = 5 * (12/13)
AP ≈ 4.615

收錄日期: 2021-05-01 09:37:09
原文連結 [永久失效]:
https://hk.answers.yahoo.com/question/index?qid=20200929150239AArBFSb







