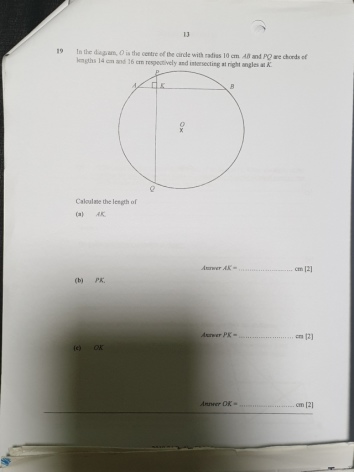
O is the centre of the circle with radius 10cm.AB and PQ?

Refer to the figure below.
Join OB , OQ and OK.
Draw OM ⊥ AB, and draw ON ⊥ PQ.

Radii: OB = OQ = 10 cm

Since O is the centre and OM ⊥ AB, AM = MB = 14/2 cm = 7 cm
Similarly, PN = NQ = 16/2 cm = 8 cm

In ΔOMB:
OM² + MB² = OB²  (Pythagorean theorem)
OM² + (7 cm)² = (10 cm)²
OM² = 51 cm²
OM = √51 cm

In ΔONQ:
ON² + NQ² = OQ²  (Pythagorean theorem)
ON² + (8 cm)² = (10 cm)²
ON² = 36 cm²
ON = 6 cm

In rectangle OMKN:
OM = NK = √51 cm
MK = ON = 6 cm

AK + MK = AM
AK + (6 cm) = 7 cm
AK = 1 cm

PK + NK = PN
PK + (√51 cm) = 8 cm
PK = (8 - √51) cm ≈ 1.12 cm

In ΔOMK:
OK² = OM² + MK²
OK² = (√51 cm)² + (6 cm)²
OK² = 87 cm²
OK = √87 cm ≈ 9.33 cm

The answers:
AK = 1 cm
PK = (8 - √51) cm ≈ 1.12 cm
OK = √87 cm ≈ 9.33 cm

 In the diagram, O is the center of the circle with radius 10 cm. 
 AB and PQ are chords of lengths 14 cm and 16 cm respectively 
 and intersecting at right angles at K. 
 Calculate the length of 
 (a) AK 
      AK = CM
 (b) PK 
      PK = 
 (c) OK
      OK =