csc (2θ) = cot(θ) + tan(θ) / 2
PLEASE HELP
Verify the identity. Assume all quantities are defined. ?
2020-04-06 1:32 pm
回答 (3)
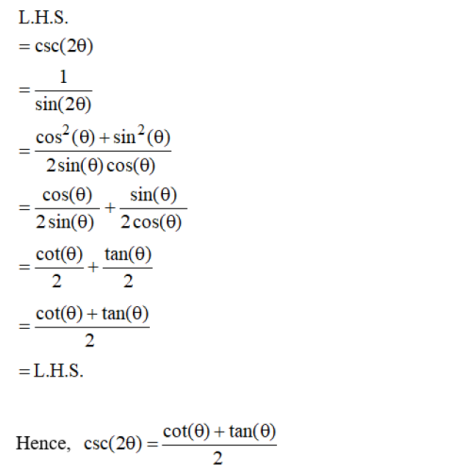
2020-04-06 2:21 pm
csc(2θ) = cot(θ) + tan(θ)
Starting with the ‘right hand side'
Right-hand side:
[ cot(θ) + tan(θ) ] / 2
= [ cos(θ)/sin(θ) + sin(θ)/cos(θ) ] / 2
= [ ( cos²θ + sin²θ ) / ( sinθcosθ ) ] / 2
= [ ( cos²θ + sin²θ ) / ( 2sinθcosθ ) ]
= [ ( cos²θ + sin²θ ) / ( sin2θ ) ]
= 1 / ( sin2θ )
= csc(2θ)
Right-hand side equals left-hand side
Starting with the ‘right hand side'
Right-hand side:
[ cot(θ) + tan(θ) ] / 2
= [ cos(θ)/sin(θ) + sin(θ)/cos(θ) ] / 2
= [ ( cos²θ + sin²θ ) / ( sinθcosθ ) ] / 2
= [ ( cos²θ + sin²θ ) / ( 2sinθcosθ ) ]
= [ ( cos²θ + sin²θ ) / ( sin2θ ) ]
= 1 / ( sin2θ )
= csc(2θ)
Right-hand side equals left-hand side
2020-04-06 5:54 pm
RTP: csc(2t) = [cot(t) + tan(t)]/2, ie., RTP: 2csc(2t) = cot(t) + tan(t)...(1).
Put (s,c) = [sin(t),cos(t)], (S,C) = [sin(2t), cos(2t)].
Then (1) becomes RTP: (2/S) = (c/s) + (s/c)...(2).
Now sc[LS(2)] = (2sc/S) = 1.
sc[RS(2)] = c^2 + s^2 = 1.
Therefore LS[(2)] = [RS(2)] & QED.
Put (s,c) = [sin(t),cos(t)], (S,C) = [sin(2t), cos(2t)].
Then (1) becomes RTP: (2/S) = (c/s) + (s/c)...(2).
Now sc[LS(2)] = (2sc/S) = 1.
sc[RS(2)] = c^2 + s^2 = 1.
Therefore LS[(2)] = [RS(2)] & QED.
收錄日期: 2021-04-24 07:50:50
原文連結 [永久失效]:
https://hk.answers.yahoo.com/question/index?qid=20200406053218AAG3F3x