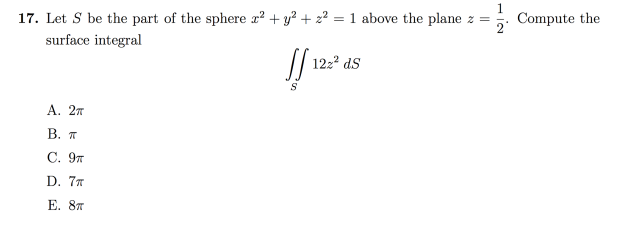
Help with surface integral?

z^2 = 1 - (x^2) - (y^2);

(x^2) + (y^2) = 1 - (1/4);

(x^2) + (y^2) = 3/4;

int_0^(2*pi) int_0^(sqrt(3)/2) 12*(1 - (r^2))*r/sqrt(1 - (r^2)) dr dθ = 7*pi;

int_0^(2*pi) int_0^(sqrt(3)/2) 12r*sqrt(1 - (r^2)) dr dθ = 7*pi

You find S as z = sqrt(1 - (x^2) - (y^2)) because that surface and not z = 1/2 because
that is what is stated.

It is stated that S:{(x^2) + (y^2) + (z^2) = 1};

We will let x = r*cos(θ); y = r*sin(θ);

(r^2) + (z^2) = 1 -> z = sqrt(1 - (r^2)) and we only care about the top part because
that is what is stated.

Now we shall put this into vector form

vector(S) = r*cos(θ) x(hat) + r*sin(θ) y(hat) + sqrt(1 - (r^2)) z(hat), therefore we can use surface
integral formula dS = |∂vector(S)/∂r × ∂vector(S)/∂θ|

∂vector(S)/∂r = cos(θ) x(hat) + sin(θ) y(hat) + -r/sqrt(1 - (r^2)) z(hat);

∂vector(S)/∂θ = -r*sin(θ) x(hat) + r*cos(θ) y(hat) + 0 z(hat);

dS = r/sqrt(1 - (r^2)) dr dθ

∫∫s 12z² dS
= ∫∫ 12z² √(1 + (∂z/∂x)² + (∂z/∂y)²) dA
= ∫∫ 12(√(1 - x² - y²))² √(1 + (-x/√(1 - x² - y²))² + (-y/√(1 - x² - y²))²) dA,
since z = √(1 - x² - y²) due to being above z = 1/2
= ∫∫ 12(1 - x² - y²) √[1 + (x² + y²)/(1 - x² - y²)] dA
= ∫∫ 12(1 - x² - y²) √[1/(1 - x² - y²)] dA
= ∫∫ 12(1 - x² - y²) dA/√(1 - x² - y²)
= ∫∫ 12√(1 - x² - y²) dA.

Since the region in the xy-plane is bounded by x² + y² + (1/2)² = 1
<==> x² + y² = 3/4 = (√3 / 2)², converting to polar coordinates yields

∫(θ = 0 to 2π) ∫(r = 0 to √3/2) 12√(1 - r²) * (r dr dθ)
= 2π ∫(r = 0 to √3/2) 12r(1 - r²)^(-1/2) dr
= 24π * -(1 - r²)^(1/2) {for r = 0 to √3/2}
= 24π * (1 - 1/2)
= 12π.

I hope this helps!