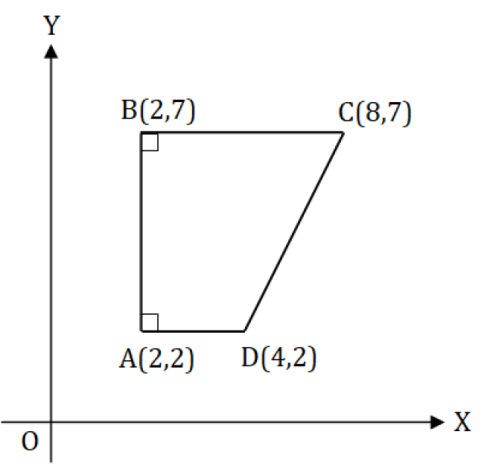
find the area of the polygon with vertices of A(2, 2), B(2, 7), C(8, 7), and D(4, 2)?

The polygon is a trapezium.
Upper base = 8 - 2 = 6
Lower base = 4 - 2 = 2
Height = 7 - 2 = 5

Area of the polygon
= (1/2) × (6 + 2) × 5
= 20 (square units)

If you graph the points, you should see you have a right trapezoid.

The bases are 6 and 2.
The height is 5

The formula for the area of a trapezoid is:
A = ½(b₁ + b₂) * h

Basically it is the average of the bases times the height.
A = ½(6 + 2) * 5
A = 4 * 5
A = 20 sq. units

If you are just given arbitrary points for the polygon and don't want to bother figuring out the shape, use the method in the following link.
A (2,2) --> 2*7 - 2*2 = 10
B (2,7) --> 2*7 - 8*7 = -42
C (8,7) --> 8*2 - 4*7 = -12
D (4,2) --> 4*2 - 2*2 = 4
A (2,2)

|10 - 42 - 12 + 4| / 2
= |-40| / 2
= 40/2
= 20

Answer:
20 sq. units