物理電路問題 求解?

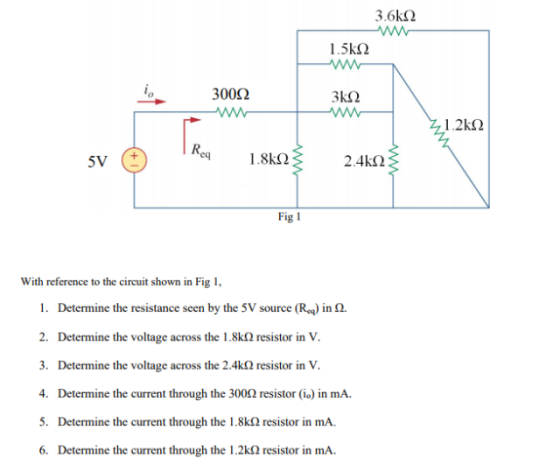
In the given circuit diagram, the 1.5k and 3k resistances are in parallel and their equivalent resistance R1, say, is in series with the equivalent resistance R2, say, of 2.4k and 1.2 k in parallel.

Here, R1 = 1.5 x 3/(1.5 + 3) k-ohm = 1 k-ohm
R2 = 2.4 x 1.2/(2.4+1.2) k-ohm = 0.8 k-ohm
Hence, equivalent resistance of the branch Re = R1 + R2 = (1+0.8) k-ohms = 1.8 k-ohms

The circuit now reduces to three resistances, namely 1.8k, 1.8k (i.e. Re above) and 3.6k, in paralle. These 3 parallel resistances are connected in series with the 300 ohms resistor.

1. The equivalent resistance RE,say, of the above 3 parallel resistnaces
= 1/(1/1.8 + 1/1.8 + 1/3.6) k-ohms = 0.72 k-ohm
Hence, Req = (0.3 + 0.72) k-ohms = 1.02 k-ohms

2. Voltage across 1.8k equals volatage acroee RE, since the two are in parallel,
Hence, V(1.8k) = 5 x (RE/Req) = 5 x (0.72/1.02) v = 3.529 v

3. Voltage across Re equals V(1.8k) since the two are in parallel,
hence, voltage across R2 = 3.529 x [R1/(R1+R2)] = 3.529 x [0.8/(1+0.8)] v = 1.568 v

4. io = 5/Req = 5/1.02 mA = 4.902 mA

5. From (2) above, voltage across 1.8k resistance = 3.529 v
Hence, current = 3.529/1.8 mA = 1.96 mA

6. Voltage across 1.2k resistance = voltage across R2(= 0.8k)
Hence, V(1.2k) = 1.568/1.2 mA = 1.31 mA